A, E, I, V, R and Ohms (And P and W)
Alphabet Soup and Math. Not your favorites, I know. I didn’t get all this at once, but I’ll share some things that helped me immensely.
First, let’s sort out these letters. Circuits have properties, and we use values to quantify those properties. There are different symbols used depending on whether we’re talking about the properties or the values, and it’s confusing when we mix two symbols interchangeably (as often happens). An engineer I respect once said, “you never use a symbol for a quantity in a formula when you are actually solving for a variable.” That might be considered a finer point by some, but he’s right. When we work formulas, we use variables. We need to use the correct symbol representing that variable.
Variables
Electromotive Force or EMF: The force that causes current to flow. It’s a difference in charge or potential between two points and it’s this difference that pushes electrons from a point of high potential to a point of low potential. The symbol for EMF is “E,” and we measure this force in volts using the symbol “V.” You’ll also see “mV” when using millivolts and “kV” for kilovolts.
Intensity: The quantity of current flowing per second through a point on a conductor. The symbol for intensity is “I” and we measure this using Amperes or Amps for short. The symbol for Amps uses “A” and you’ll often see “mA” for milliamps.
Resistance: The opposition to the flow of current and the symbol is “R.” Each part of a circuit offers resistance, even the wire itself. We measure this in ohms and use the omega symbol, Ω. You’ll often see something like “2k Ω” to represent 2,000 ohms.
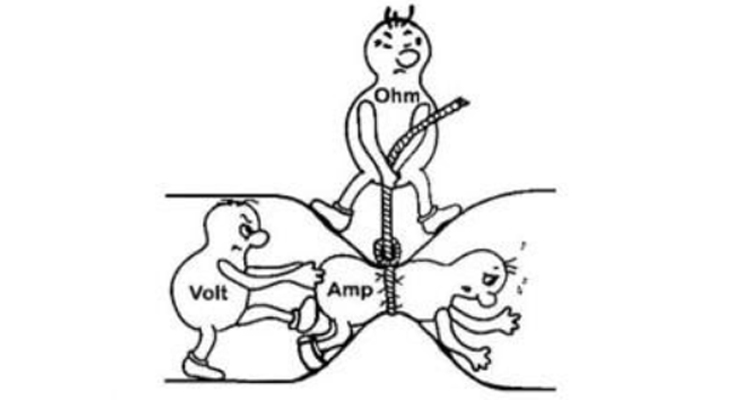
A popular illustration I’ve found helpful to understand this relationship between voltage, current and resistance is this one:
 Impedance: DC (direct current) circuits have resistance. AC (alternating current) circuits have resistance but also capacitive and inductive reactance that oppose the changes of voltage and current in the AC waveform. The amount of reactance is frequency-dependent, and although we sometimes substitute Z for R in the formulas, we aren’t really calculating for impedance; we can’t measure impedance using a common multimeter. The symbol used for Impedance is “Z,” and like resistance, we measure this in ohms and use the omega symbol, Ω.
Impedance: DC (direct current) circuits have resistance. AC (alternating current) circuits have resistance but also capacitive and inductive reactance that oppose the changes of voltage and current in the AC waveform. The amount of reactance is frequency-dependent, and although we sometimes substitute Z for R in the formulas, we aren’t really calculating for impedance; we can’t measure impedance using a common multimeter. The symbol used for Impedance is “Z,” and like resistance, we measure this in ohms and use the omega symbol, Ω.
Power: Related to Ohm’s Law, it refers to the amount of work done in a circuit. We use the symbol “P.” We measure this in watts using the symbol “W.” You’ll also see “mW” for milliwatts or “kW” for kilowatts. The by-product of this work is heat, and that can be a problem if we don’t manage the heat well in a rack or other equipment enclosure.
Ohm’s Law Formula
So how do I remember the Ohm’s Law formula? “Basic Electricity” by Van Valkenburgh, Nooger and Neville, Inc. It was originally written back in 1954 and introduced me to “The Magic Triangle:”
 E, I and R. Ohm’s Law. How do I use this Magic Triangle? Put your finger over the variable you wish to solve for and take a look at what’s left. Want to solve for E? With your finger over E; what’s left looks like I times (x) R. Solving for I? That looks like E over R or E divided by (÷) by R. The same with solving for R. That’s E divided by (÷) I.
E, I and R. Ohm’s Law. How do I use this Magic Triangle? Put your finger over the variable you wish to solve for and take a look at what’s left. Want to solve for E? With your finger over E; what’s left looks like I times (x) R. Solving for I? That looks like E over R or E divided by (÷) by R. The same with solving for R. That’s E divided by (÷) I.
What’s a practical application? Let’s say I have a device that requires 12 V DC, and the power supply provided with the device is rated at 13.5 V DC and provides 0.5 A. To make things look tidy, I wish to remove the power supply 65 feet away and I’ll be using 24 AWG wire. Recall that the wire has resistance, and I need to calculate the voltage drop across the wire. Voltage drop is simply referring to the loss of electrical pressure as current flows through a resistance. The goal is to see if I’ll still have the necessary 12 V at the end of the 65-foot wire run.
What do I know? Let’s find out:
The power supply provides 0.5 A of current, and a common brand 24 AWG stranded copper wire has 0.026 ohms per foot. While the power supply is 65 feet away, it’s actually 130 feet of wire. Why? There and back, plus and minus of the circuit – it’s two wires, not just one. 0.026 per foot x 130 feet = 3.38 ohms of resistance total in this wire run.
Let’s solve for E, the voltage drop, across the wire.
- E = I x R
- E = 0.5 A x 3.38 Ω
- E = 1.69 V
I started with 13.5 V from the power supply minus the 1.69 V that I’m losing in the 130 feet of 24 AWG wire. 13.5 minus (-) 1.69 leaves me with only 11.81 V for a device needing 12 V. Oops. My choices are to either shorten the wire run or use a larger AWG wire.
If I use 22 AWG wire at 0.0165 ohms per foot (0.0165 per foot x 130 feet = 2.145 ohms):
- E = 0.5 A x 2.145 Ω
- E = 1.0725 V
13.5 V minus (-) the 1.0725 voltage drop across the wire leaves me with 12.43 volts for the device. The 12 V device will be happy and life will be good. No service call needed!
Power as related to Ohm’s Law has its own “Magic Triangle:”
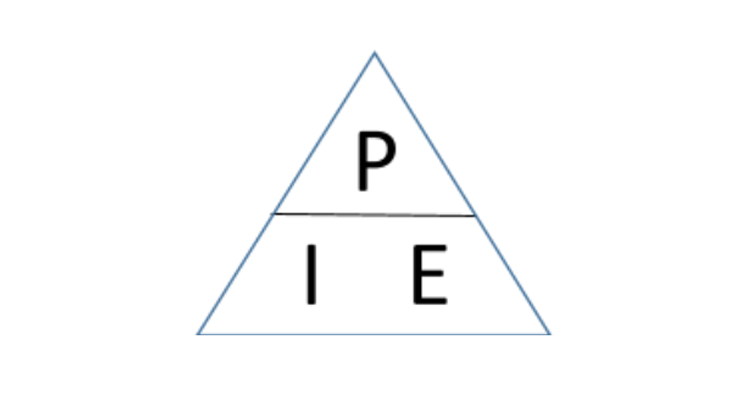 Some would say “it’s as easy as PIE” or “P = I x E.” Just like the Ohm’s Law triangle, place your finger over the variable you wish to solve for:
Some would say “it’s as easy as PIE” or “P = I x E.” Just like the Ohm’s Law triangle, place your finger over the variable you wish to solve for:
- P = I x E
- I = P / E
- E = P/I
We use this frequently to calculate the current draw for equipment. Let’s say that we have two new 7,000 lumen 4K projectors going into a classroom. I want to know if I can put both on the same 20 A circuit, but I need to know how much current each projector will draw. The specs say the max power consumption is 1,070 W. I put my finger over the I and the formula looks like I = P / E.
What do I know? Let’s find out:
- P = 1,070 W from the spec.
- E = 120 V is the mains power here in the US
- I = P / E
- I = 1,070 W / 120 V
- I = 8.92 amps
8.92 x 2 projectors = 17.84 A. Even though it may be a 20 A circuit, the NEC tells me that I can’t put more than a 16 A continuous load on a 20 A circuit. Since projectors are essentially big light bulbs, I would consider them continuous loads and almost 18 A is over the 16 A allowance. I’ll need to put the projectors on separate 20 A circuits.
This even works for hair dryers (and the heat guns we use with heat shrink tubing). Let’s say I buy a new, 1,200 W hairdryer. What’s the current draw?
- I = P / E
- I = 1,200 W / 120 V
- I = 10 A
My new 1,740 W heat gun would draw 14.5 A
- I = P / E
- I = 1,740 W / 120 V
- I = 14.5
So, if you plug in a second hair dryer or heat gun on the same circuit and try and use both simultaneously, the result will be a tripped circuit breaker.
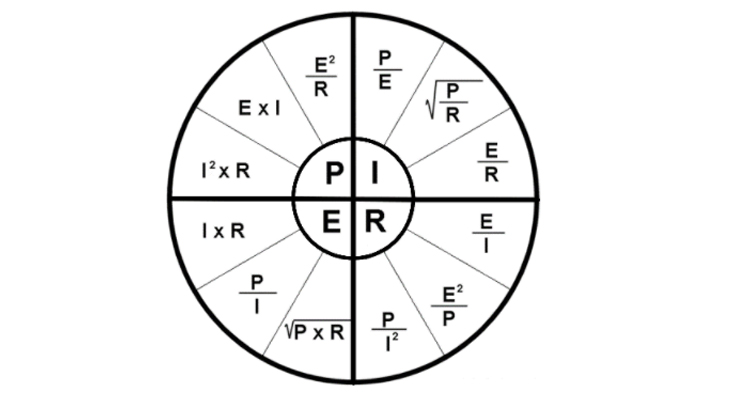
Formula Wheel
Having the entire formula wheel, containing formulas for solving all four properties is certainly handy:
 But if you don’t have the wheel, the EIR and PIE triangles are easy to memorize and use. Here are some references that have been helpful to me:
But if you don’t have the wheel, the EIR and PIE triangles are easy to memorize and use. Here are some references that have been helpful to me:
- Fujitaki, K. (2009). “The Manga Guide to Electricity.” No Starch Press.
- Van Valkenburgh, Nooger, Neville. (1992). “Basic Electricity.” Prompt Publications.
- Holt, M. (2004). “Basic Electrical Theory.” Mike Holt Enterprises, Inc.
- Legrand AV. “Optimized Power Distribution and Grounding for Audio, Video and Electronic Systems”
Hopefully, this has energized your understanding of Ohm’s Law and Power and how it is all related together.







